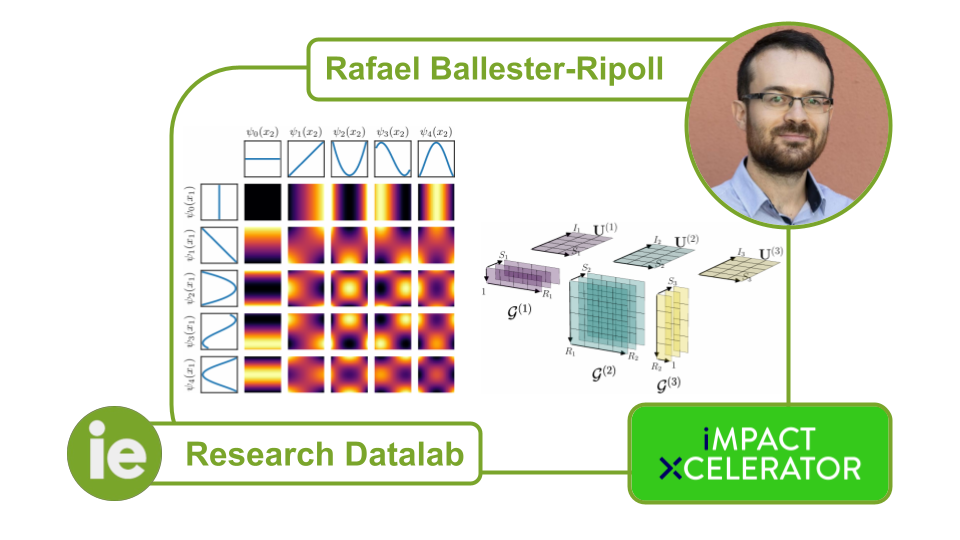
IE Research Datalab is proud to announce that professor Rafael Ballester-Ripoll has published the paper “Computing Statistical Moments Via Tensorization of Polynomial Chaos Expansions” in the Journal on Uncertainty Quantification.
Rafael’s research introduces a novel algorithm for estimating complex statistical moments of multidimensional functions represented by polynomial chaos expansions (PCE). The algorithm cleverly decomposes the PCE into a low-rank tensor network, which simplifies the process and significantly speeds up calculations. Using benchmark engineering functions, the study demonstrates that this method is not only faster but also maintains a minimal approximation error compared to other algorithms. Moreover, it can adapt to changes in input variable distributions without the need for retraining, making it highly efficient and versatile.
In essence, professor Ballester-Ripoll has developed a way to quickly and accurately analyze the behavior of systems with many variables, which is common in engineering and risk assessment. By transforming the PCE into a more manageable form, Rafael has opened the door to a variety of numerical methods that can now be applied more effectively. This advancement holds promise for improving sensitivity analysis, global optimization, and other tasks that require handling high-dimensional functions, potentially leading to more precise predictions and better-informed decisions in various scientific and engineering fields.
Read the paper here: https://doi.org/10.1137/23M155428X